Rock Deformation

17 minutes read

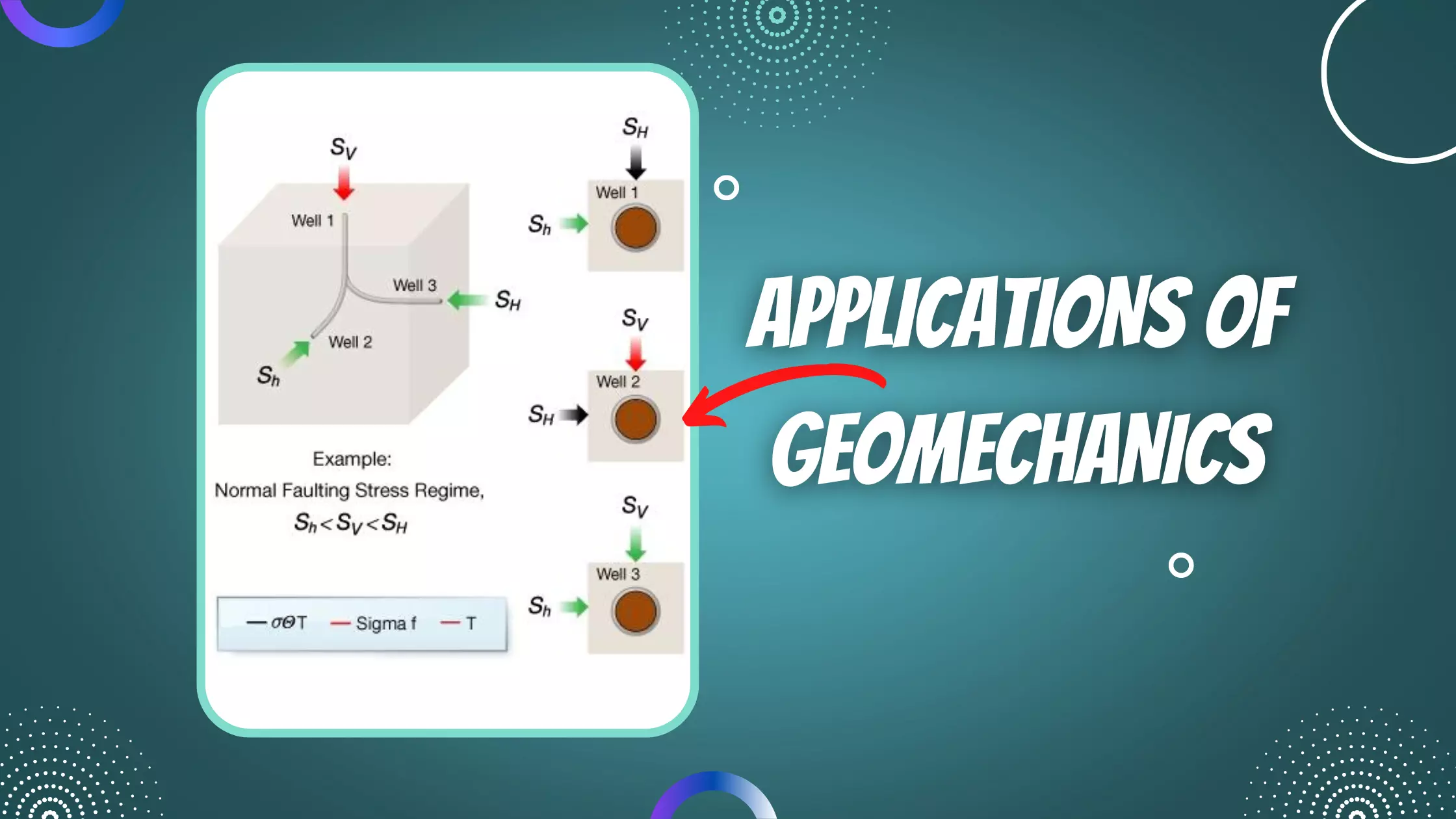
Ductile Processes

Ductile rocks respond to stress by flowing. Production-induced pressure changes can trigger ductile flow of reservoir rock (Figure 1). When the reservoir rock flows, massive solids production can occur, killing production from a well and generating high workover costs. Therefore, it is useful to know whether a rock in the subsurface is deforming in a brittle or ductile manner.

**Figure 1**: Ductile rocks respond during drilling

Ductile deformation develops at relatively high effective mean stress and is characterized by large non-recoverable, or plastic, strain $\varepsilon _p$ (Figure 2).

Viscoelastic-plastic response — **Figure 2**: Ductile deformation

As the effective mean stress on a rock increases, the rock response to loading undergoes a transition from localized brittle failure to a more distributed ductile mode of deformation. This change in the mode of deformation is called the brittle-ductile transition (Figure 3).

Cataclasis and the Brittle-Ductile Transition

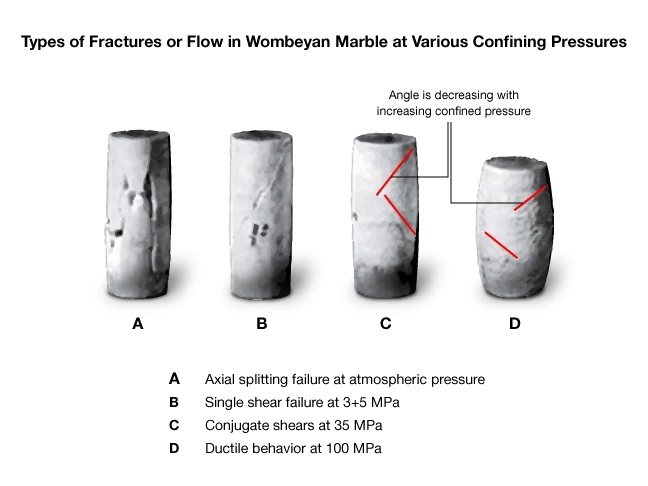
The brittle–ductile transition coincides with the onset of cataclastic flow. Cataclastic deformation occurs when brittle fracture and frictional processes are active simultaneously. On a Mohr stability diagram, cataclastic flow develops when Amonton’s criterion intersects Coulomb’s or Mohr’s shear failure criterion. Cataclastic flow is characterized by microfracturing of mineral grains and frictional sliding along particle boundaries. Compare the brittle deformation in cores A and B with the ductile deformation of cores C and D (Figure 3).

Stress-strain response of Wombeyan marble

A: At atmospheric pressure, the sample deforms by axial splitting, such as, $\beta$ ~90 degrees.
B: As the confining pressure increases from 3 to 5 MPa, a discrete shear fracture develops.
C: At a confining pressure of 35 MPa, conjugate shear fractures have formed and a distinct barreling of the sample can be seen and $\beta$ ~45 degrees. The material is now at the brittle-ductile transition.
D: The sample is fully ductile at 100 MPa confining pressure.

At low confining pressures, the rock fails by brittle fracture. When the rock fractures, there is an abrupt drop in the load carrying capacity after peak stress. This is known as strain softening.
As confining pressure increases, peak axial stress increases and the load carrying capacity beyond peak stress increases. This is called the residual strength, which is governed by the frictional strength of the newly created shear fracture(s).
As the confining pressure increases further, the magnitude of axial strain increases and the stress drop disappears (peak stress minus residual stress). When the confining pressure is great enough, rocks deform by flow. That is, the rock deforms without loss of axial stress and with little volumetric strain. This is the brittle-ductile (B-D) transition pressure.
At confining pressures greater than that B-D transition, the load carrying capacity of the rock may increase. This is known as strain hardening.

Beyond the brittle ductile transition, the distinct barrel shape and the absence of discrete shear fractures is evidence of deformation that is homogeneously distributed throughout the sample at the microstructure level. Notice that the angle $\beta$ decreases as confining pressure increases. This decrease in $\beta$ indicates that the friction angle is decreasing with increasing mean stress, consistent with Mohr’s failure criterion but not Coulomb’s. This evolution from brittle-to-ductile behavior is characteristic of all sedimentary rocks ranging from very high-strength, low-porosity sandstones and limestones to high porosity rocks and mechanically compacted clays. The main difference from one type of rock to another is the state of stress over which the transition occurs.

Grain Crushing and Shear Enhanced Compaction

Grain crushing is an important deformation mechanism that operates under hydrostatic stress. It is particularly important in high porosity rocks where sand production may be an issue. Unlike failure criteria previously mentioned, grain crushing occurs without shear stress and so the grain-crushing criterion plots as a point on the normal stress axis of Mohr’s stability diagram.

The phenomenon of grain crushing results from stress concentrations at grain-to-grain contacts. In 1990, Zhang et al. used Hertzian contact theory to show that the hydrostatic grain crushing pressure is a function of porosity and grain size. They showed that for rocks with a given grain size, the higher the porosity the lower the grain crushing pressure. Alternatively, for rocks with a given porosity, the larger the grain size the lower the grain crushing pressure. This prediction enables one to use geologic knowledge of depositional environment and grain-size to predict variations in reservoir strength. Figure 5 shows the dependence of grain crushing pressure on the product of porosity and grain size.

**Figure 4**: Conceptual model of grain contact stresses, $\sigma _r$ , subjected to hydrostatic stress $P$

Grain crushing can also contribute to rock deformation under differential stress. In this case the effective mean stress need only reach the grain crushing pressure. Such a mode of deformation is called shear-enhanced compaction. Shear-enhanced compaction can be expected in high-porosity reservoirs with initially high pore pressure. Simply reducing pore pressure can lead to grain crushing.