Reservoir Engineering | Basic Concepts in Reservoir Engineering

42 minutes read

Reservoir simulation, Fluid flow, Petrophysics, Formation evaluation, Well testing, Enhanced oil recovery, Geostatistics, Pore pressure prediction, Porosity, Permeability, Saturation, Well performance, Well logging, Reservoir characterization, Reservoir management, Reservoir modeling, Production optimization, Water flooding, Gas reservoirs, Oil reservoirs, Reservoir monitoring, Reservoir analysis, Well spacing, Reservoir performance, Reservoir geomechanics, Basic concepts of reservoir engineering, Basic concepts of civil engineering, What is reservoir engineering, 3 types of reservoir, Reservoir engineering basics, Reservoir engineering pdf, Reservoir engineering handbook pdf, Basic concepts of environmental engineering

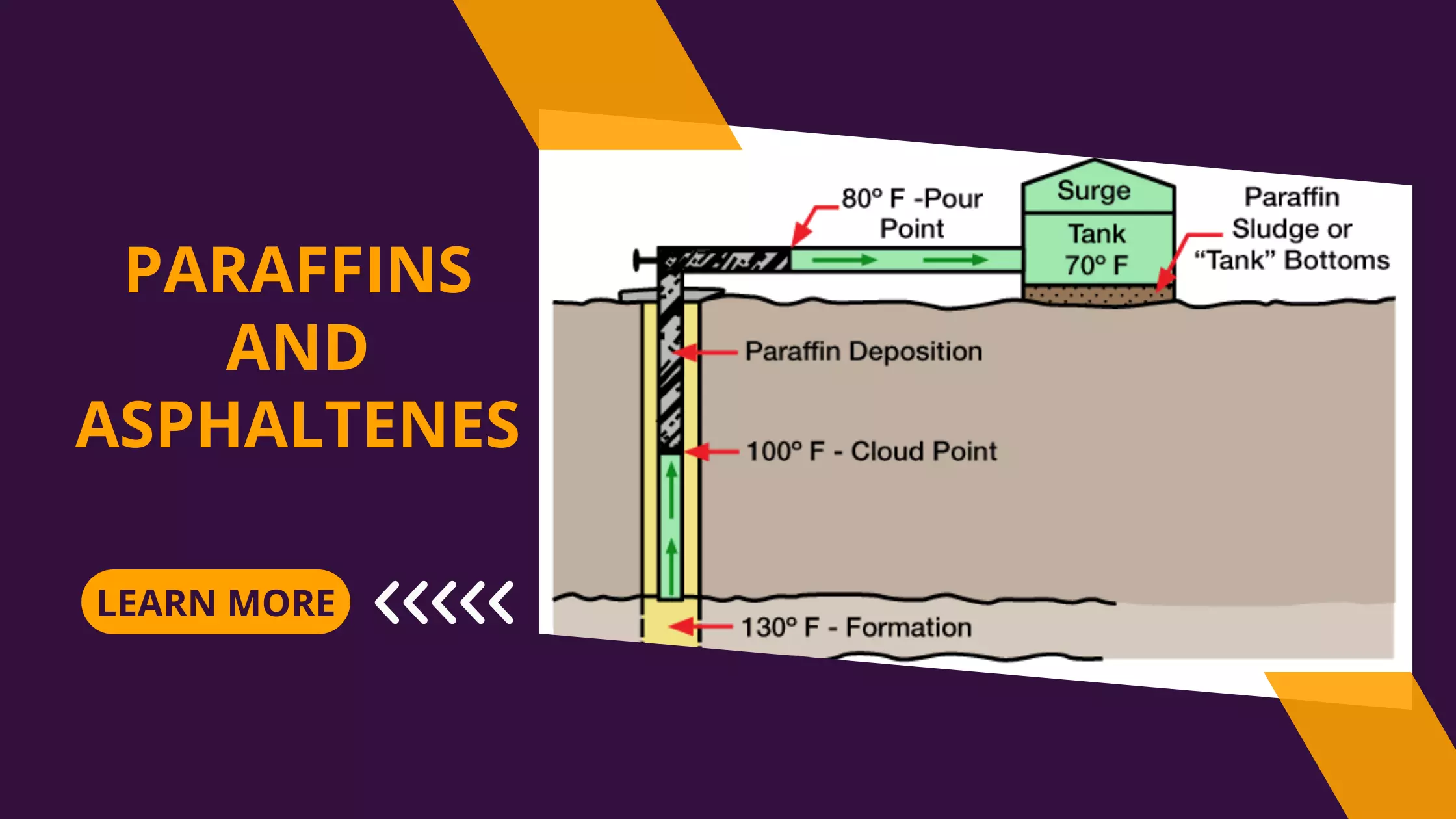
Pressure and Temperature

Reservoir pressure is one of the most important parameters of reservoir engineering calculations. Whether the calculations involve the tank type model or a more sophisticated reservoir simulator, accurate pressure values are required. However, there is an important difference between the requirements of the two models. The unit tank model relies on material balance equation calculations, and requires the average pressure for the whole reservoir as a function of time or production. In reservoir simulation studies, however, it is strongly desirable to have available buildup pressure values for individual wells as a function of time. These values represent the average pressure for the drainage volumes of the wells, and are needed for the history-matching phase of the simulation study, which is performed to validate the accuracy of the model built to represent the reservoir (Matthews et al. 1954). History matching is an essential step in “tuning” a reservoir model before conducting a predictive study.

Reservoir engineering calculations require a value for the pressure in the reservoir, away from the wellbore. To obtain this value, the well must be shut in and the pressure increase with shut-in time must be recorded. We refer to this as a pressure buildup test (Matthews and Russell 1967). From these data the average pressure value is calculated.

Another way of obtaining average values is to record the pressure in a well in which Production has been suspended. if such a well exists, and it is not very close to a producer or an injector, a pressure-measuring device can be used to continuously record the pressure, without interrupting production or injection operations.

For the single-tank model, an average value for the whole reservoir is required. This is normally obtained by a volumetric averaging of the pressure values from different wells. The equation for this purpose is

$\overline{p}_{R}=\dfrac{\displaystyle\sum \, {p_{i}}V_{i}}{\displaystyle\sum V_{i}}$ ………… (3)

where:

$\overline{p}_{R}$ = average pressure for reservoir

$p_{i}$ = average pressure for Well i

$V_{i}$ = the drainage volume of Well i

Thus, if there are three wells with pressures p₁, p₂, and p₃, and drainage volumes V₁, V₂, and V₃, then Equation 3 becomes:

$\overline{p}_{R}=\dfrac{p_{1}\, V_{1}+p_{2}\, V_{2}+p_{3}\, V_{3}}{V_{1}+V_{2}+V_{3}}$

Matthews et al. (1954) and Matthews and Russell (1967) have shown that the well-drainage volume V₁ is proportional to its flow rate, q₁ Substituting q_ifor V_i in Equation 3 gives

$\overline{p}_{R}=\dfrac{\displaystyle\sum p_{i}\ q_{i}}{\sum q_{i}}$ ………… (4)

Equation 4 is the more Practical equation because the flow rate is usually available, while it may be more difficult to estimate the drainage volume.

A very useful plot is that of the average pressure values obtained on several wells versus the total oil production of an oil reservoir, or total gas production of a gas reservoir. The pressures are plotted on the Y-axis. If there is continuity in the reservoir the Pressures from the various wells should plot close to each other. If the pressures for a well plot are consistently higher or lower than the other values, it may indicate that the well is not in good communication with the reservoir or that it is in a separate reservoir. This may point out the need for more wells to effectively drain the isolated portion of the reservoir. Furthermore, the data from the isolated well should not be lumped in with the data from other wells in material balance engineering calculations.

Before comparing the pressure values measured in wells at various depths in a reservoir (very thick and/or steeply dipping reservoirs), they should be referred to a datum depth (Figure 1).

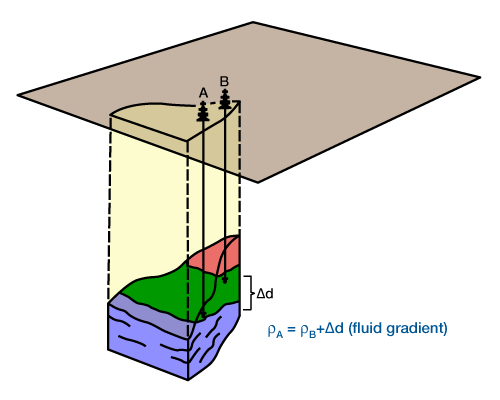
datum depth, Reservoir simulation, Fluid flow, Petrophysics, Formation evaluation, Well testing, Enhanced oil recovery, Geostatistics, Pore pressure prediction, Porosity, Permeability, Saturation, Well performance, Well logging, Reservoir characterization, Reservoir management, Reservoir modeling, Production optimization, Water flooding, Gas reservoirs, Oil reservoirs, Reservoir monitoring, Reservoir analysis, Well spacing, Reservoir performance, Reservoir geomechanics, Basic concepts of reservoir engineering, Basic concepts of civil engineering, What is reservoir engineering, 3 types of reservoir, Reservoir engineering basics, Reservoir engineering pdf, Reservoir engineering handbook pdf, Basic concepts of environmental engineering — **FIGURE 1** Datum depth

Normally the depth of the volumetric midpoint of the reservoir is taken as the datum depth. This is determined by constructing a plot of depth versus cumulative pore volume (Figure 2).

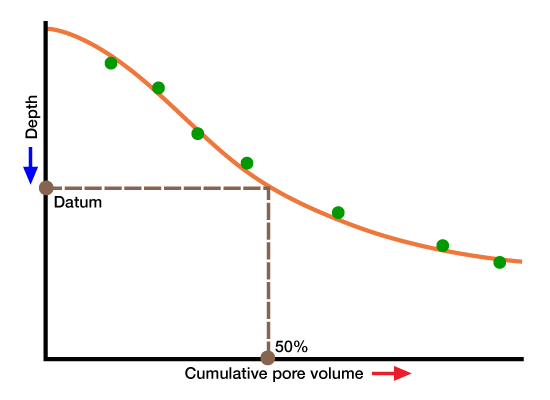
plot of depth versus cumulative pore volume, Reservoir simulation, Fluid flow, Petrophysics, Formation evaluation, Well testing, Enhanced oil recovery, Geostatistics, Pore pressure prediction, Porosity, Permeability, Saturation, Well performance, Well logging, Reservoir characterization, Reservoir management, Reservoir modeling, Production optimization, Water flooding, Gas reservoirs, Oil reservoirs, Reservoir monitoring, Reservoir analysis, Well spacing, Reservoir performance, Reservoir geomechanics, Basic concepts of reservoir engineering, Basic concepts of civil engineering, What is reservoir engineering, 3 types of reservoir, Reservoir engineering basics, Reservoir engineering pdf, Reservoir engineering handbook pdf, Basic concepts of environmental engineering — **FIGURE 2** Plot of depth versus cumulative pore volume

The depth corresponding to 50% pore volume is the volumetric midpoint depth. If a particular pressure value is obtained at a different depth than the datum, it is adjusted to the datum by

$p_{adj} = p + 0.433\, \gamma \, \Delta H$ ………… (5)

$p_{adj} = p - 0.433\, \gamma \, \Delta H$ ………… (6)

where:

p = the pressure at any elevation, psi

$\gamma$ = specific gravity of fluid

ΔH = the vertical distance between the point at which the pressure was measured and the datum depth, ft

Equations 5 and 6 apply when the point at which the Pressure was determined is, respectively, above and below the datum depth.

When an aquifer is associated with the reservoir, the Pressure behavior as a function of time at the hydrocarbon-water contact (or as close as possible to it) is needed for water influx calculations. If this is not available, one usually uses the average reservoir Pressure and adjusts it to the hydrocarbon-water contact depth.

The average reservoir pressure is needed in many reservoir engineering calculations. In the case of miscible EOR techniques, for example, the average reservoir pressure determines whether miscibility will occur when CO₂ or other gases are injected. This in turn affects overall recovery and the economic feasibility of the project.

Reservoir pressure is a topic of significance in reservoir engineering because it is one of the critical pieces of data required by the reservoir engineer for an effective analysis of a reservoir. obtaining reliable pressure data should be a primary goal of any reservoir management program.

Reservoir Temperature

The calculation of primary recovery relies on the reasonable assumption that the reservoir temperature stays constant. Thus, hydrocarbon recovery during this phase is considered to be an isothermal process. This is so because as fluids are Produced any change in temperature due to Production is compensated for by heat from the cap or base rocks, which are considered to be heat sources of infinite capacity.

The average reservoir temperature is needed for laboratory analyses that are made at reservoir conditions. Determining fluid properties, such as viscosity, density, formation volume factor, and gas in solution, requires a value for reservoir temperature. Reservoir temperature is usually measured at the bottom of the well or wells in a reservoir using a wireline temperature gauge. If a variation in temperature is detected across a reservoir after correcting for depth, an average value can be used for the constant reservoir temperature.

For EOR techniques such as chemical and miscible processes, temperature affects the phase behavior of injected and produced fluids, and thus the recovery. The feasibility of these processes must be determined by laboratory tests carried out at reservoir temperature. In EOR processes that employ heat injection, such as steam or in-situ combustion, the reservoir temperature is not constant and hydrocarbon recovery is not an isothermal process. Therefore, in mathematical formulations of such processes, it is necessary to write an energy balance over the entire reservoir. From an operations standpoint, reservoir temperatures need to be measured continuously at monitoring wells. These measurements indicate the heat front’s pattern of movement. Normally, a uniform movement is desired, but the heat-front pattern can be altered by changes in injection and/or production schedules.